Laboratory for Flow Control-Fluid mechanics experiment for science & technology-

Development and application of USR

“Rheology is science of material deformation without considering fluid flows.” Some researchers have explained so. This is because torque rheometer, which is the standard tool to evaluate rheological properties of fluids, assumes ideal shear conditions in a test fluid layer. To keep the conditions the layer is required to be thin enough, around 1 mm, but there are multiple factors that make the conditions to be unsatisfied in testing general materials, for example, slip on the sensing walls, shear banding, elastic instability, and heterogeneity of test materials.

　We, LFC researchers and students, have investigated bubble suspension rheology in the framework of studies on bubbly drag reduction, and noticed that there is no suitable tool to explore the bubble rheology. Idea of ultrasonic spinning rheometry (USR) was thus invented along our research philosophy. Velocity-profiling-assisted rheometer had been temporarily introduced to solve the problem of imperfect shear conditions in the torque rheometer, but USR completely stands apart from these methods.
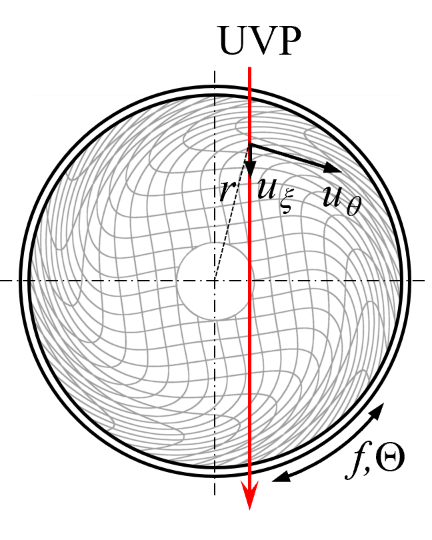
Principle: USR utilizes an open top cylindrical container filled with a test fluid (see the figure below) in periodic oscillations with a certain frequency and an amplitude. The oscillation of the cylinder wall thus propagates toward interior of the test fluid depending on local rheological properties. Shear rate distributes in the radial direction of the cylinder, and thus time variations of instantaneous radial velocity profiles reflect the rheological properties. If we can assume ideal one-directional, axisymmetric flow in the azimuthal direction, for the fluid flow in the cylinder, the flow is dominated by Cauchy’s equation of motion,

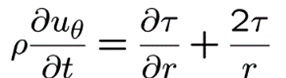
and constitutive equations,

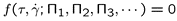
The flow field in the cylinder should be a solution of the equations above, and in principle, substituting the velocity information measured by a tool to the equations can determine the rheological properties. In USR, ultrasonic velocity profiling (UVP) is utilized to acquire the velocity information. UVP is originally one-directional one-component velocity profile measurement in the propagation line of ultrasonic wave. Thanks to axisymmetry of the flow field, radial profiles of azimuthal velocity component are obtained by a simple data conversion with a displacement of the measurement line from the center of the cylinder (see the figure).

Phase differential method [1]: USR has now two major algorithms to evaluate rheological properties and “phase differential method” is the first method that pioneered USR. In usual velocity profile measurement, the velocity data includes considerable measurement errors. Substituting these directly into the equations of motion induces augmentation of the errors by differential scheme on the equations. The method utilizes local phase information of the flow oscillation extracted by Fourier transform. Radial gradient of the phase is determined by viscosity in cases of Newtonian fluids. Local effective viscosity is thus determined by comparing the phase gradient with that obtained from the exact solution of the oscillation flow. Radial profile of the effective shear rate is also obtained from velocity information measured by UVP. And, quasi-instantaneous viscosity curve, which can represent shear-dependent viscosity, is given as the results of the analysis.

Linear viscoelastic analysis [2]: Effective viscosity evaluated by the phase differential method explained above reflects elasticity in addition to viscosity in applications to viscoelastic materials. Linear viscoelastic analysis was thus proposed to access more detailed information of viscoelasticity. We adopt local Maxwell model,

where the viscosity 𝜇 and elasticity E allow to vary in the radial direction depending on the local shear rate. To avoid augmentation of the measurement errors, analyses are performed on frequency domain, namely, the velocity data, equation of motion, and the local Maxwell model are Fourier-transformed as,

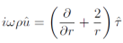
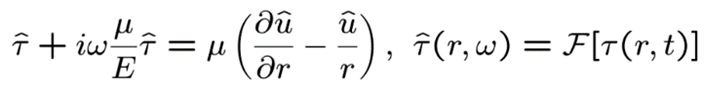
Viscosity and elasticity are determined as values to minimize the following cost function

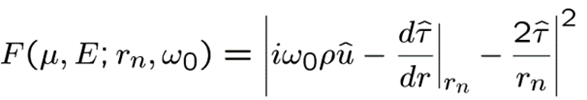
In actual calculation, phase of linear viscoelasticity,

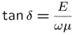
is explored instead of the elasticity.

Applicability and applications: Regarding the principle of USR, advantages of USR can be summarized as:

1. Applicability for heterogeneous and multiphase fluids
2. Quasi-instantaneous evaluation of the rheological properties
3. Quantification of fluid characteristics observed on their flows
4. Evaluation of unsteady shear effect

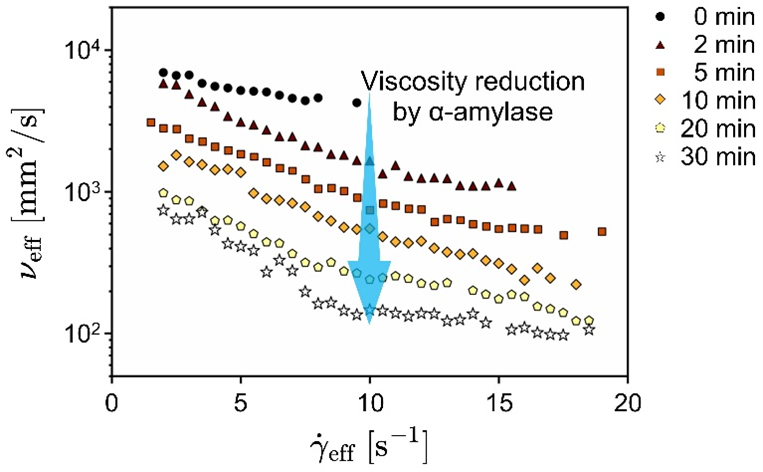
Figure 2 shows time variations of quasi-instantaneous viscosity curves representing influence of hydrolysis of rice gruel by added alpha amylase. Applicable range of USR for viscosity is determined by viscous layer thickness. Considering with measurable range of UVP, it should be from O(1 mm²/s) to O(10³ mm²/s) in kinematic viscosity. It means that USR are complementarily used with standard torque rheometers. Efficacy of the methods were confirmed [3,4] and there are many published applications:

1. suspensions of bubbles, particles, and drops [1,2,5,6,7]
2. foods (see the figure) [3,8,9,10]
3. complex polymer solutions [4,11,12]

Rheological properties evaluated here reflect actual unsteady fluid flows formed in the cylindrical vessel. The properties therefore should be used to reproduce and predict unsteady flows in any configuration. In the project of PRESTO-JST, we will open up the frontier of flow prediction and control for complex fluids based on the rheological properties obtained with USR [12].

　The idea of USR has been applied industrial field and novel measurement principle and tools were invented, for example, an idea of non-invasive in-line rheometry[13] and carriable USR[14].

References

1. Y.Tasaka, T.Kimura, Y.Murai “Estimating the effective viscosity of bubble suspensions in oscillatory shear flows by means of ultrasonic spinning rheometry” Exp. Fluids, 56 (2015) 1867.
2. Y.Tasaka, T.Yoshida, R.Rapberger, Y.Murai “Linear viscoelastic analysis using frequency-domain algorithm on oscillating circular shear flows for bubble suspensions” Rheol. Acta, 57 (2018) 229–240.
3. T.Yoshida, Y.Tasaka, Y.Murai “Rheological evaluation of complex fluids using ultrasonic spinning rheometry in an open container” J. Rheol., 6 (2017) 537-549.
4. T.Yoshida, Y.Tasaka, Y.Murai “Efficacy assessments in ultrasonic spinning rheometry: Linear viscoelastic analysis on non-Newtonian fluids” J. Rheol., 63 (2019) 503-516.
5. T.Yoshida, Y.Tasaka, Y.Murai “Effective viscoelasticity of non-Newtonian fluids modulated by large-spherical particles aligned under unsteady shear” Phys. Fluids, 31 (2019) 103304.
6. T.Yoshida, Y.Tasaka, P.Fischer, Y.Murai “Time-dependent viscoelastic characteristics of montmorillonite dispersion examined by ultrasonic spinning rheometry” Applied Clay Science, 217 (2022) 106395
7. K.Ohie, T.Yoshida, H.J.Park, Y.Tasaka, Y.Murai “Evaluation on time variation of effective viscosity by ultrasonic spinning rheometry (Application to separating oil-water mixture)” Trans. JSME, 86 (2020) 20-00242.
8. T.Yoshida, Y.Tasaka, P.Fischer “Ultrasonic spinning rheometry test on the rheology of gelled food for making better tasting dessert” Phys. Fluids (Special topic: Food and Fluids), 31 (2019) 113101.
9. K.Ohie, H.Chiba, S.Kumagai, T.Yoshida, Y.Tasaka “A method for evaluating time-resolved rheological functionalities of swallowed foods” J. Texture Studies, 53 (2022) 445-452.
10. A.Takano, K.Ohie, Y.Horimoto, Y.Murai “Evaluation of time-dependent rheological property in the coagulation of skimmed milk by ultrasonic velocity profiler” Trans. JSME, 88 (2022) 22-00115.
11. K.Ohie, T.Yoshida, Y.Tasaka, Y.Murai “Effective rheology mapping for characterizing polymer solutions utilizing ultrasonic spinning rheometry” Exp. Fluids, 63 (2021) 40.
12. K.Ohie, T.Yoshida, Y.Tasaka, M.Sugihara-Seki, Y.Murai “Rheological characterization and flow reconstruction of polyvinylpyrrolidone aqueous solution by means of velocity profiling-based rheometry” Exp. Fluids, 63 (2022) 135.
13. Y.Tasaka, T.Yoshida, Y.Murai “Non-intrusive in-line rheometry using ultrasonic velocity profiling”, Ind. & Eng. Chem. Res., 60 (2021) 11535–11543.
14. T.Yoshida, K.Ohie, Y.Tasaka “In situ measurement of instantaneous viscosity curve of fluids in a reserve tank” Ind. & Eng. Chem. Res., 61 (2022) 11579 – 11588.